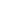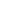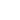1.2.2 John G. Simmons Formula in a Case of Small, Intermediate and High Voltage (Field Emission Mode).
According chapter 1.2.1, the approximate expression for the tunneling current in the MIM system can be written as [1]:
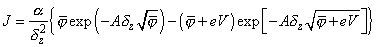
(1)
where
 ,
,
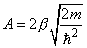 ,
,
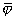 – average barrier height,
– average barrier height,
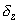 – barrier width,
– barrier width,
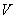 – voltage between electrodes.
– voltage between electrodes.
Small voltage.
At low voltages
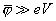 , expression (1) can be simplified [1]
, expression (1) can be simplified [1]
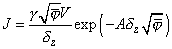
(2)
where
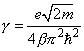 . Since
. Since
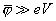 , we can consider that
, we can consider that
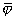 doesn't depend on
doesn't depend on
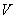 . Thus, in the case of small applied voltage, the tunneling current proportionate to
. Thus, in the case of small applied voltage, the tunneling current proportionate to
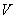 . Energy diagram of the MIM system then
. Energy diagram of the MIM system then
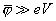 is shown on Fig. 1.
is shown on Fig. 1.
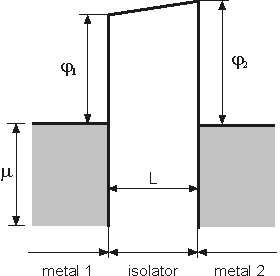
Fig. 1. Potential barrier in the MIM system then
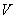 ~ 0.
~ 0.
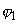 and
and
 – work function of the left and right metals, respectively.
– work function of the left and right metals, respectively.
Intermediate voltage.
If
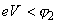 , then
, then
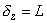 and
and
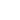
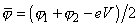 (Fig. 2).
(Fig. 2).
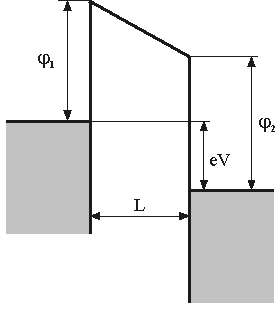
Fig. 2. Potential barrier in the MIM system then
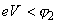 .
.
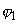 and
and
 – work function of the left and right metals, respectively.
– work function of the left and right metals, respectively.
In [2] it is shown, that for this case the tunneling current-voltage relation is given by
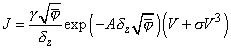
(3)
where
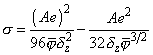 .
.
High voltage – Field emission mode.
The case when
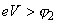 corresponds to energy diagram shown in Fig. 3 and to the following
corresponds to energy diagram shown in Fig. 3 and to the following
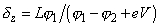 ,
,
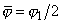 .
.
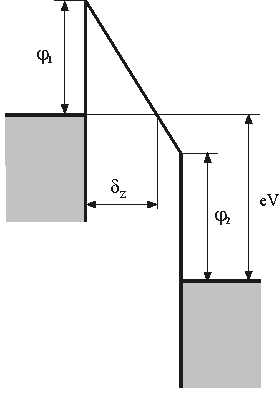
Fig. 3. Potential barrier in the MIM system then
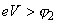 .
.
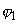 and
and
 – work function of the left and right metals, respectively.
– work function of the left and right metals, respectively.
Substituting
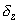 and
and
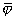 into equation (1), we obtain
into equation (1), we obtain
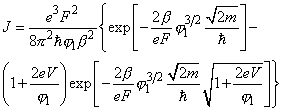
(4)
where
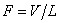 – electric field strength.
– electric field strength.
At high applied voltage (
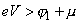 ) the Fermi level of electrode 2 is lower than the conduction band bottom of electrode 1. Under such conditions, electrons can not tunnel from electrode 2 into electrode 1 because of lack of empty states. An inverse situation is for electrons tunneling from electrode 1 into empty states of electrode 2. This process is similar to autoelectronic emission from a metal into vacuum. Thus, since
) the Fermi level of electrode 2 is lower than the conduction band bottom of electrode 1. Under such conditions, electrons can not tunnel from electrode 2 into electrode 1 because of lack of empty states. An inverse situation is for electrons tunneling from electrode 1 into empty states of electrode 2. This process is similar to autoelectronic emission from a metal into vacuum. Thus, since
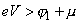 , the second summand in (4) can be neglected and for the current we get
, the second summand in (4) can be neglected and for the current we get
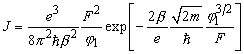
(5)
where coefficient b = 23/24. This result agrees qualitatively with an analytical expression for the field emission current density [3].
Thus, using formulas (2)–(5), we can compute the tunnel current at given system parameters and plot current-voltage characteristics. Fig. 4 shows theoretical tunneling current-applied voltage plot in case of carbon electrode 1
(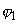 = 4,7 эВ) and platinum electrode 2
(
= 4,7 эВ) and platinum electrode 2
( = 5,3 эВ) at
= 5,3 эВ) at
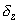 = 5 Å and contact area S = 10-17 m2.
= 5 Å and contact area S = 10-17 m2.
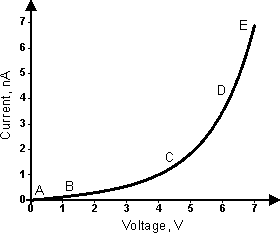
Fig. 4. Current-voltage characteristic for carbon electrode 1 and platinum electrode 2 at
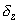 = 5 Å and contact area 10-17 m2. Parts of J(V) curve correspond to the following expressions: AB – (22), BC – (23), CD – (24), DE – (25).
= 5 Å and contact area 10-17 m2. Parts of J(V) curve correspond to the following expressions: AB – (22), BC – (23), CD – (24), DE – (25).
Summary.
- Depend upon magnitude of applied voltage, formula (1) can be simplified (2)–(5).
- It is possible to describe the experimental tunneling current dependences by approximated expressions (2)–(5) in accordance with magnitude of applied voltage.
References.
- John G. Simmons. J. Appl. Phys. - 1963. - V. 34 1793.
- John G. Simmons. J. Appl. Phys. - 1963. - V. 34 238.
- Dobretzov L.N., Gomounova M.V. Emission electronics. Nauka, 1966 (in Russian)