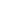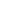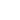Phase-distance curves
Amplitude-modulation (intermittent-contact, semicontact) mode is widely spreaded oscillating mode and generally speaking can be interpreted and described by the amplitude, phase, frequency and dissipation on one another or on the cantilever-sample distance dependences.
The study of such dependences is necessary according to following circumstances. First of all it relates to obtaining high-grade images (without noise and with high resolution). Then the study of suitable dependences can help in determining the nature of tip-sample interaction, defining forces included in this interaction and formation SPM images.
At last the study of suitable curves can help in obtaining more contrastive images and quantitative parameters of sample under investigation. Obtained in Amplitude-modulation mode images are determined by the row of factors related to the sample as well as to conditions of measurement and values of scanning parameters.
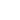
For interpreting results of amplitude-modulation mode usage one can to study dependence on the tip-sample distance the phase lag between cantilever oscillations and driving force. Suitable phase-distance (p-d) curves (their typical view one can see on the animated picture) can be monotonic or can to have areas of bistability and hysteresis. The presence of the bistability (as is shown on the same picture) leads to arising of the artifacts on the images obtained in Amplitude-modulation mode. Origin of bistability lies in possibility of simultaneously co-existence oscillations predominantly in attractive or predominantly in repulsive potentials.
The bistability also can arise in complicated shape of tip-sample potential when in initial area cantilever stiffness is greater than potential derivative and the potential derivative becames greater than cantilever stiffness.
With suitable choise of set-point amplitude of cantilever oscillation, its stiffness, sharpness of the tip one can reach conditions when over all sample surface under investigation areas with bistability are absent.
References
- Phys. Rev. B 60, 4961 (1999).
- Surface Sciece 460, 292 (2000).
- Phys. Rev. B 66, 041406 (2002).